Physics Puzzler: Swinging Physics

Pendulums are objects swinging periodically from a fixed support in a gravitational field. A simple pendulum is a negligibly small object called a bob, suspended by a string of negligible weight from a fixed point. Its period is given by T = 2π√(R/g), where R is the length of the suspension string and g is the acceleration due to gravity. This equation is good in the approximation of small angles of swing.
But the idealized swinging point mass is a bit unrealistic. Increase the size of the bob and you get a slightly different result. If the swinging object is of considerable size, or the angle of swing very large, the result can be very different.
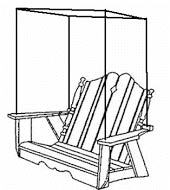
A swing with four parallel ropes supporting it. Author image.
One example of a nonsimple pendulum is the very familiar porch swing: a chair, usually seating two people, suspended from the ceiling by four ropes. Let’s consider the simplest type in which the four ropes are of equal length and all parallel, attached between the chair arms and the ceiling. By itself, it has a natural period, T, swinging in the usual way, front to back. The formula above applies, but only if you insert an appropriate value for the suspension length, R. Should this be
a. the length of the supporting ropes?
b. the distance from the ceiling support to the chair seat?
c. the average of the above two distances?
d. the sum of the rope lengths?
Suppose Galileo—an aficionado of the pendulum—and his daughter Maria Celeste sit on the swing, enjoying the relaxing front-to-back motion, back and forth. What is the period of this motion now?
a. greater than the unloaded swing
b. the same as the unloaded swing
c. less than the unloaded swing
Now suppose that our esteemed Italian scientist and his daughter are bored with swinging forward and backward. They decide to swing sideways. What is the period of the motion now?
a. greater than the unloaded swing
b. the same as the unloaded swing
c. less than the unloaded swing
Ready for the answers? See “Swinging Physics: The Answer



